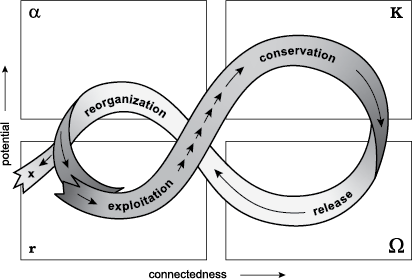
Resilience is an emergent property of systems - and is the amount of disturbance a system can absorb before it crosses a critical threshold and reorganizes. Reorganization can return a system to its original state (undergoing an adaptive cycle, see right), or the system can reorganize into a different state.
Frequently, new states of systems are not desirable, because humanity adapted to the systems we inhabit and relies on a relatively predictable suite of ecological goods and services, which changes when a system reorganizes into something new. An example of an undesirable reorganization following collapse is the sudden onset of the Dustbowl following relatively slow landscape change and drought. The recovery of the landscape affected by the Dustbowl also provides a positive example of transformation - the purposeful erosion of the resilience of a system in an undesirable state and human-mediated transformation to a more desirable state.
Ecological resilience and engineering resilience are often used interchangeably but are very different. Engineering resilience (also called resiliency or bounceback), is the rate of return of a system following disturbance. This is sometimes a useful metric, and is included in the concept of ecological resilience, but bounceback fails to account for thresholds in systems (it assumes a system can always bounceback given sufficient time), and the fact that systems are always changing (i.e., are non-stationary).
Resilience Science
Crawford Stanley Holling is considered the father of resilience research and crucial to the establishment of resilience research centers around the world. Holling highlighted the importance of considering surprise, system reorganization, and learning when trying to understand social-ecological dynamics. These efforts lead to new ideas about the dynamic nature of resilience and the co-development of Adaptive Environmental Management and Assessment, a learning-based approach to the management of complex environmental problems.
Examples of Resilience

Grasslands
A significant threat exemplifying why resilience matters, is the invasion of Nebraska grasslands by woody species and subsequent change of grassland habitat to woodland or forest habitat. Such a change reflects a loss of resilience of the grassland and the emergence of an alternative “state” of the former grassland. This example of alternative states (grassland to woodland) exemplifies the type of broad change that CRAWL is focused on understanding and preventing. For grasslands, conversion to woodland reduces the value of these lands to livestock, negatively affecting livelihoods in a state where beef production is an important economic driver. Furthermore, when grasslands change to woodlands, changing woodlands back to grasslands is non-trivial; woodlands have high resilience and are difficult to transform back to grassland (i.e., hysteresis). Furthermore, it can be more difficult to change back than it was to change in the first place - the “path in” is not the same as the “path out”.
Row Crops
Many systems in Nebraska have already undergone undesirable change, and in this case, management seeks to reduce the impacts, or transform the system back to a more desirable state. The Dustbowl is a recent historic example of a desirable state shifting to an undesirable system state (from grassland to Dustbowl). The Dustbowl also illustrates the idea of transformation - active human-guided regime change, from an undesired state to a desired state. Former Dustbowl lands are now some of the most productive in the US.

Rivers
The Platte River Basin is a significant resource for Nebraska and the Great Plains. It provides drinking water, irrigation waters for agriculture, and habitat for wildlife, including migratory and endangered species. The Platte formerly existed in a braided, sand-dominated highly mobile state, dominated by the effects of spring floods that scoured sandbars of vegetation. Water control structures and needs have altered flows and limited flood events, leading to an alternative river state dominated by stationary islands, incised channels, and vegetated sand bars. This state is desirable for humans (because it best provisions irrigation waters and constant flows) but undesirable for many wildlife species.
Lakes
A classic example of alternative stable states is the change in shallow lakes from clear water to algal-dominated. This type of regime change occurs in Nebraska lakes. In some of our lakes, the algal-dominated state of the lakes is dominated by toxic algae, making the undesired state of these lakes especially undesirable.
Resilience Models
Below are competing models representing the resilience response of systems over time and to perturbations. In A, B, and C, resilience is shown in terms of hypothetical system trajectory and is shown on the Y-axis and time on the X-axis. In D, E, and F resilience is considered from a complex adaptive systems point of view.
A. A stationary system without perturbation.
System trajectory does not change or vary.
B. A stationary single equilibrium system with perturbation.
System trajectory drops with perturbation but bounces back with time. Here, the only metric is the time required to bounce back to equilibrium. Use of this model could lead to the erroneous conclusion that all systems will recover given sufficient time
C. A stationary single equilibrium system with an alternative configuration of trajectory.
This model, as with Grafton et al. Figure 1, fails to capture the potential for systemic changes between regimes that lead to completely different trajectories following perturbation.
D. Ball and cup diagram of alternative states (cups) in a non-stationary non-equilibrium system without perturbation.
This shows the state of the system (circle), which emphasizes its complex adaptive nature, rather than a specific system structure.
E. Ball and cup diagram of alternative states in a non-stationary non-equilibrium system with perturbation.
In this case, perturbation does not exceed the resilience of the system. System trajectories are expected to vary but are maintained within a single basin of attraction (i.e., it has adaptive capacity conferred by ecological stability measures).
F. Ball and cup diagram of alternative states in a non-stationary non-equilibrium system with perturbation that exceeds the resilience of the system.
The system is moved into an alternative basin of attraction, with completely different system-level properties (performance, function, structures, processes, and feedbacks).
